How do we introduce students to the meaning of argumentation? What can be some discipline-specific considerations in doing so?
Pedagogical Strategies
Introducing Argumentation to Science Teachers: Questioning and Public Recording of Ideas
In this video clip, Professor Sibel Erduran brainstorms about the meaning of argumentation in her teaching of in-service science teachers who are participating in a part-time Master’s programme in Teaching and Learning. She begins the class by asking questions about the meaning of argumentation and records the participant responses on the white board thereby making a public representation of the ideas generated in class. The brainstorming of participants’ ideas is particularly important in order to gain understanding of where they are coming from. The pedagogical strategies utilised are ‘questioning’ and ‘brainstorming and understanding student ideas’.
Emphasising appropriate strength of claims and evidence for a field/discipline
In this video segment, Dr Liam Guilfoyle is introducing a literature review task that students will undertake in the field of educational research. Referring to previous readings that the group had discussed, he uses the students’ own critiques of the papers to focus on the way in which arguments are being presented within these published pieces. He highlights, through comparison, the tentative versus bold nature of claims and the forms of qualifiers sometimes used by authors nuance their claims.
This inducts students into the specific norms of arguments within a discipline or field, but also opens the space for critique of arguments based on how convincing they are to the students as readers. Finally, the students are encouraged to consider what they find convincing when reading literature as educational practitioners and to emulate this in their construction of arguments, rather than necessarily following ‘formulas’ for constructions.
Scaffolding reflection on one’s own and other’s arguments
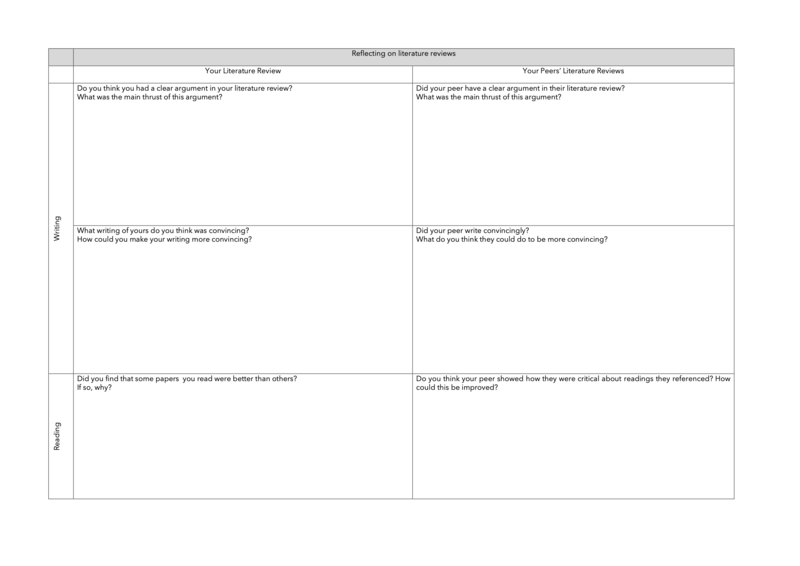
In this video, Dr Liam Guilfoyle provides a brief overview an example scaffold for supporting students to reflect on the arguments in their own writing. They are also asked to critique their peers writing for quality of argument, which is an important skill in its own right but used here for the purpose of comparison and inward reflection. The mirrored columns of the reflection sheet is designed to give students the opportunity to reflect inwards the critiques they can see in others’ work.
Creating a Need for Learning about Argumentation that meets Disciplinary Standards in Mathematics
In mathematics, a large body of research documents a discrepancy between common student notions about argumentation and notions of argumentation that are consistent with how argumentation is typically viewed in the discipline of mathematics. Indeed, research indicates that students of all levels of education (including university students) tend to believe that, by verifying the truth of a general mathematical statement in a few cases covered by the generalisation, offers enough evidence to establish the truth of the generalization.
Here’s an example of a mathematical generalisation and two typical student arguments that illustrate the common student notion of argumentation described above:
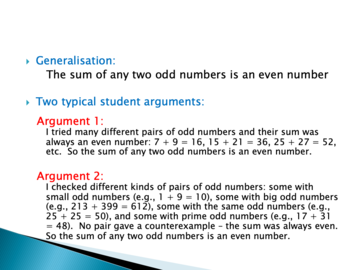
The two student arguments claim to show the truth of the generalisation by checking a few cases. However, the fact that the generalisation is true in some cases is not a guarantee that the generalization is true for all cases. So both of these arguments are invalid and do not offer conclusive evidence for the truth of the generalisation, i.e., they are not proofs. Students who propose such arguments do not see a need to learn about the kind of arguments which would meet disciplinary standards as they do not see a problem with their own argumentation practices. This is major obstacle in introducing students to the meaning of argumentation in mathematics.
How can we help these students understand the limitations of their current conceptions of argumentation and see an intellectual need (Harel, 1998) for changing those conceptions to ones that are more aligned with disciplinary practices?
This is a challenging question that requires careful instructional planning to address (for details, see Stylianides & Stylianides, 2009). One important element of this planning is to help students reflect on their current mathematical conceptions, confront contradictions that arise in situations in which some of these conceptions no longer hold, and recognise the importance (need) of modifying these conceptions to resolve the contradictions. The following slides illustrate a task used by Professor Gabriel Stylianides in his teaching to help students see an intellectual need for learning about secure methods of validation in mathematics (the task was adapted from Mason et al., 1982).
Task posed to the students (adapted from Mason et al. 1982) |
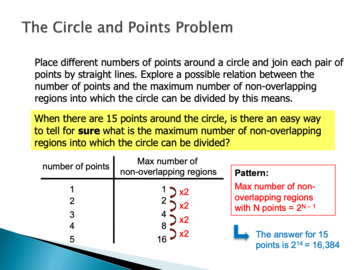
Typical student solution to the task by examining the first 5 cases in the generalisation |
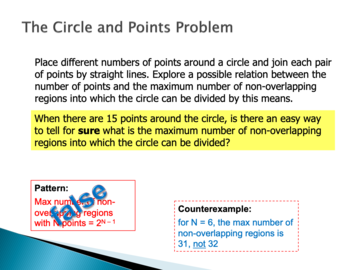
Students recognise that the generalisation is false when prompted by the instructor to check the sixth case in the generalisation |
Stylianides presents the formula that gives the correct answer to the task.
